We evaluate the line integral using the following parametrization of the circular path

$\begin{align*}\mathbf{r}(t) = \mathbf{i} R \cos t + \mathbf{j} R \sin t \end{align*}$
The derivative of 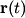$\mathbf{r}(t)$ is
$\begin{align*}\mathbf{r}(t) = -\mathbf{i} R \sin t + \mathbf{j} R \cos t \end{align*}$
So, the line integral is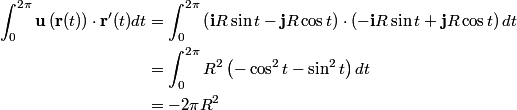
$\begin{align*}\int_{0}^{2 \pi} \mathbf{u}\left(\mathbf{r}(t)\right) \cdot \mathbf{r}'(t) dt &= \int_{0}^{2 \pi} \left(\ma...$
The problem statement does not tell us whether to traverse the circle clockwise or counterclockwise, so we assume that only the magnitude of the line integral is important. Therefore, (C) is the correct answer.

Solution to 1996 Problem 43